Ahlfors' Lemma gives the principal criterion for obtaining lower bounds
on the Kobayashi metric.
Ahlfors' Lemma 1
Let
ds2 = h(z)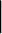 dz
dz be a Hermitian pseudo-metric on
Dr,
h∈C2(Dr), with ω the associated
(1, 1)-form. If
Ric
be a Hermitian pseudo-metric on
Dr,
h∈C2(Dr), with ω the associated
(1, 1)-form. If
Ric  ω≥ω on
Dr,
then
ω≤ωr on all of
Dr (or equivalently,
ds2≤dsr2).
ω≥ω on
Dr,
then
ω≤ωr on all of
Dr (or equivalently,
ds2≤dsr2).
Lemma 1.1 (negatively curved families)
Let
{ds12,..., dsk2} be a negatively curved family of metrics
on
Dr, with associated forms ω1, ..., ωk.
Then
ωi≤ωr for all i.
Then our main theorem:
Theorem 1.2
Let dmax and dmin be the maximum, resp. minimum distance
between any two adjacent vertices of a quadrilateral Q. Let σ
be the diagonal pigspan of a pig P with four legs.
Then P is capable of standing on the corners of Q iff
σ≥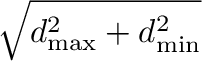 . .
|
(1) |
Corollary 1.3
Admitting reflection and rotation, a three-legged pig P is capable of
standing on the corners of a triangle T iff (![[*]](crossref.png) ) holds.
) holds.
Remark 1
As two-legged pigs generally fall over, the case of a polygon of order
2 is uninteresting.
Exercise 1
Generalize Theorem ![[*]](crossref.png) to three and four dimensions.
to three and four dimensions.
Note 1
This is a test of the custom theorem style `note'. It is supposed to have
variant fonts and other differences.
B-Theorem 1
Test of the `linebreak' style of theorem heading.
This is a test of a citing theorem to cite a theorem from some other source.
1 (Theorem 3.6 in [
1])
No hyperlinking available here yet ... but that's not a
bad idea for the future.
![\begin{proof}
% latex2html id marker 113
[Proof of Theorem \ref{pigspan}]
And another test.
\end{proof}](img6.png)
![\begin{proof}[Proof (necessity)]
And another.
\end{proof}](img7.png)
![\begin{proof}[Proof (sufficiency)]
And another.
\end{proof}](img8.png)
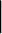 dz
dz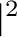 be a Hermitian pseudo-metric on
Dr,
h∈C2(Dr), with ω the associated
(1, 1)-form. If
Ric
be a Hermitian pseudo-metric on
Dr,
h∈C2(Dr), with ω the associated
(1, 1)-form. If
Ric  ω≥ω on
Dr,
then
ω≤ωr on all of
Dr (or equivalently,
ds2≤dsr2).
ω≥ω on
Dr,
then
ω≤ωr on all of
Dr (or equivalently,
ds2≤dsr2).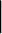 dz
dz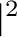 be a Hermitian pseudo-metric on
Dr,
h∈C2(Dr), with ω the associated
(1, 1)-form. If
Ric
be a Hermitian pseudo-metric on
Dr,
h∈C2(Dr), with ω the associated
(1, 1)-form. If
Ric  ω≥ω on
Dr,
then
ω≤ωr on all of
Dr (or equivalently,
ds2≤dsr2).
ω≥ω on
Dr,
then
ω≤ωr on all of
Dr (or equivalently,
ds2≤dsr2).![[*]](crossref.png) ) holds.
) holds.
![\begin{proof}
% latex2html id marker 113
[Proof of Theorem \ref{pigspan}]
And another test.
\end{proof}](img6.png)
![\begin{proof}[Proof (necessity)]
And another.
\end{proof}](img7.png)
![\begin{proof}[Proof (sufficiency)]
And another.
\end{proof}](img8.png)